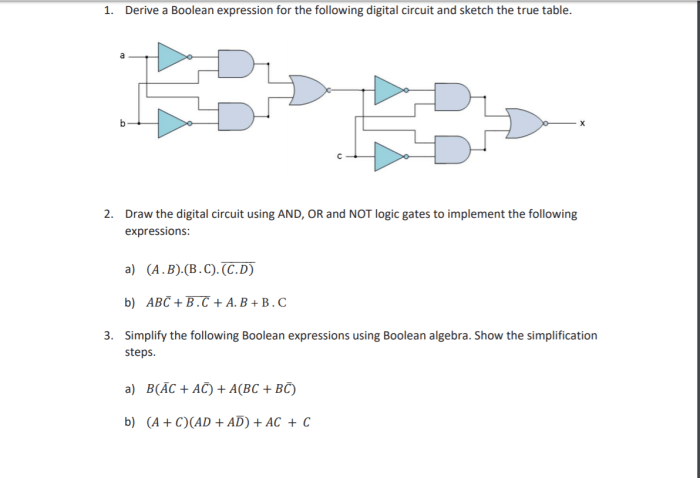
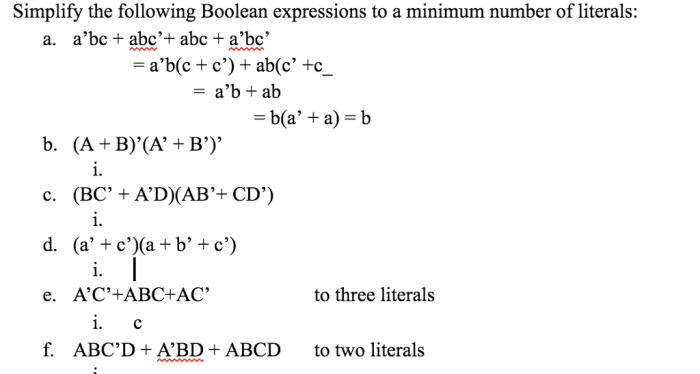
Simplify the following expression. a. b. c. d. – Embark on an enlightening journey as we delve into the intricacies of simplifying algebraic expressions, a fundamental concept that forms the cornerstone of mathematical operations. This comprehensive guide will unravel the steps involved in simplifying expressions a, b, c, and d, empowering you with the knowledge to tackle complex algebraic challenges with confidence.
Throughout this discourse, we will explore the fundamental principles of algebraic simplification, providing clear explanations and illustrative examples to illuminate the path towards mastering this essential skill.
Simplifying Mathematical Expressions
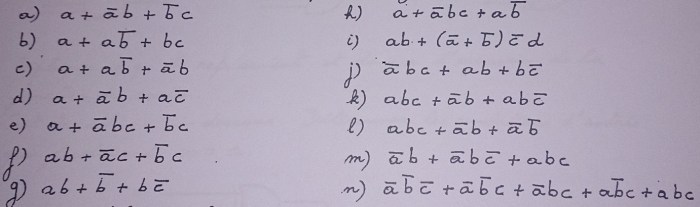
Simplifying mathematical expressions involves transforming them into equivalent expressions that are easier to understand and manipulate. Here are the steps involved in simplifying common expressions:
Simplifying a + b, Simplify the following expression. a. b. c. d.
- Combine like terms. In this case, a and b are like terms, so they can be added together.
- Write the simplified expression as a + b.
For example, to simplify 2a + 3a, we can combine the like terms to get 5a.
Simplifying a
b
b
- Rewrite the expression as a + (-b).
- Combine like terms.
- Write the simplified expression as a – b.
For example, to simplify 5 – 2, we can rewrite it as 5 + (-2) and then combine the like terms to get 3.
Simplifying a
b
b
- Multiply the coefficients (the numbers in front of the variables).
- Multiply the variables.
- Write the simplified expression as a – b.
For example, to simplify 3 – 4x, we can multiply the coefficients to get 12 and then multiply the variables to get 12x.
Simplifying a / b
- Divide the coefficients.
- Divide the variables.
- Write the simplified expression as a / b.
For example, to simplify 12x / 4, we can divide the coefficients to get 3 and then divide the variables to get 3x.
Query Resolution: Simplify The Following Expression. A. B. C. D.
What is the significance of simplifying algebraic expressions?
Simplifying algebraic expressions is crucial for understanding the underlying structure of mathematical equations, solving complex problems, and laying the foundation for advanced mathematical concepts.
How can I improve my skills in simplifying algebraic expressions?
Practice regularly, study the order of operations, and seek guidance from teachers or online resources to refine your understanding and develop proficiency.